Required
Everything that falls into a black hole is encoded on it’s surface
Hologram
Let’s quickly remember what a hologram is.
It is a 2D surface that generates a 3D scene.

The information needed to construct an object of a certain dimension can be contained in a surface one dimension lower.
Here’s a very long video explaining in depth how a 2D hologram can create a virtual 3D scene.
The optics are complicated - but basically you record the interference of the scene lighting against a reference beam that you can then use to reconstruct the scene using the same reference beam.
Foundation
- We know from Special Relativity that an object moving at 100% the speed of light experiences no time from an outside perspective.
- We know from General Relativity that objects of high mass curve spacetime in a way that moves the underlying ‘grid’
So the question arises - what happens when an object of high enough mass curves spacetime so strongly that the underlying ‘grid’ is moving faster than the speed of light?
This is of course the event horizon of a black hole.
But something else unintuitive happens because of the first point - the event horizon isn’t just a boundary where light can’t escape, it is a point where time stops from the outside perspective.
Let’s explore this by imagining watching someone else fall into a black hole. We’ll be on a spaceship far enough away where we don’t notice the spacetime curvature of the black hole.
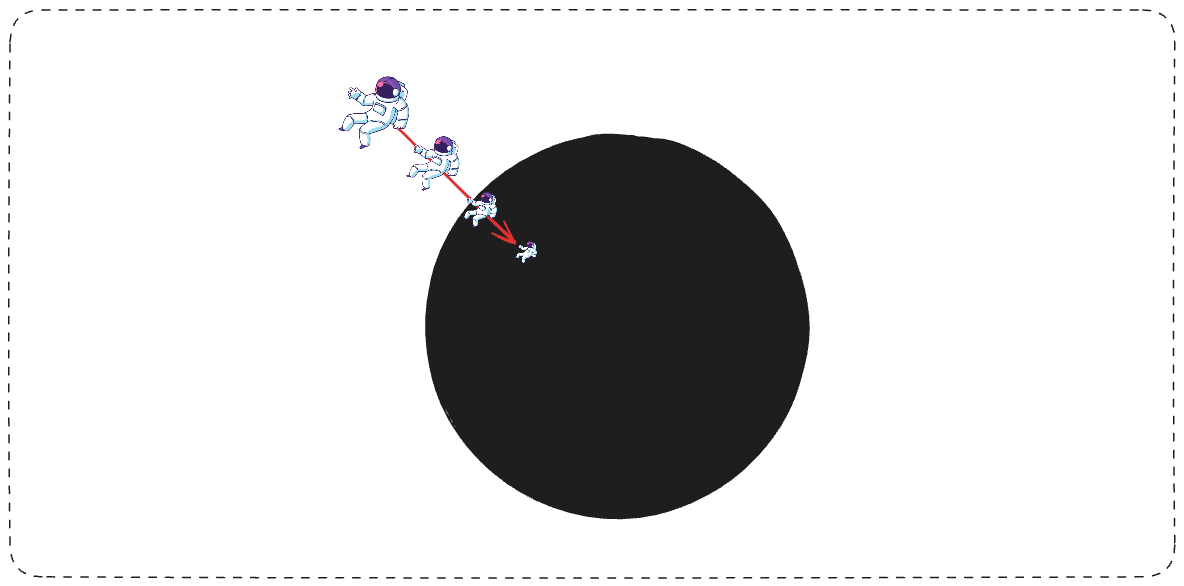
We might intuitively imagine that they would fall in like anything else. We would watch them fall faster and faster, until they cross the event horizon and poof - they disappear.
However, thanks to General Relativity, we would see something much different. As they get closer and closer to the horizon, spacetime curvature becomes more and more extreme - this transfers their temporal speed into spacial speed.
Instead of watching them speed up, we would see them start to move in slow motion. They would slow down in time.
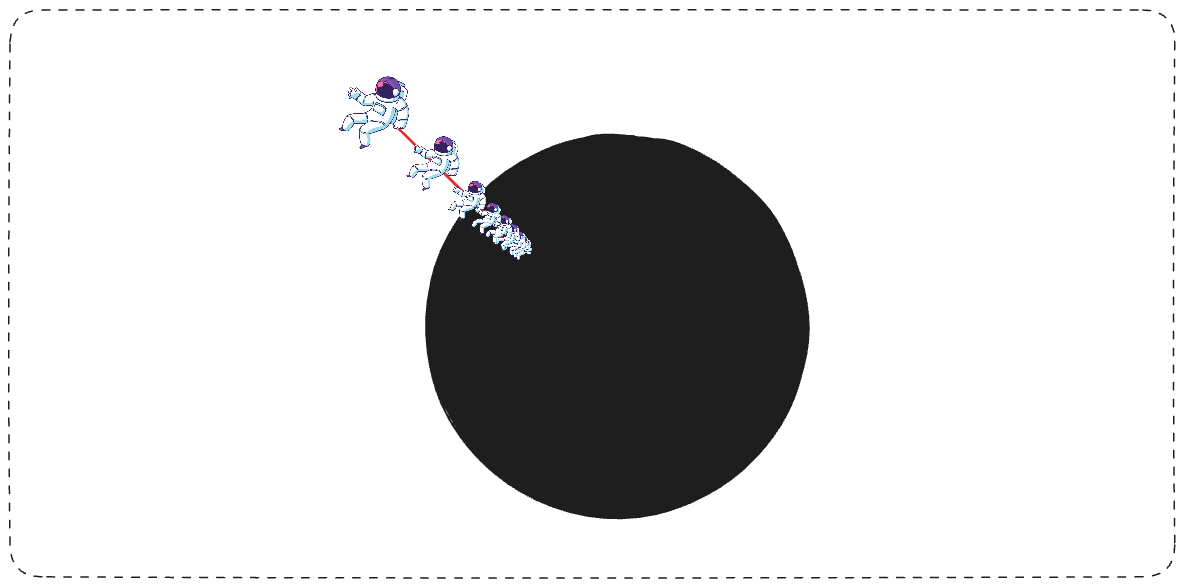
Eventually when they do hit the event horizon, we would see them stop completely in time - frozen on the surface. Any light emitted by them after crossing is trapped within the horizon.
Eventually their frozen image would be red-shifted from view. We would watch them slowly become more and more red, stopped in time, until they fade away.
Because of relativity, from the astronaut’s perspective he experiences himself falling through the horizon - time doesn’t stop for him.
This leads to a paradox.
If from our perspective they are frozen on the surface of the black hole, and from theirs they have fallen inside - where are they actually located?
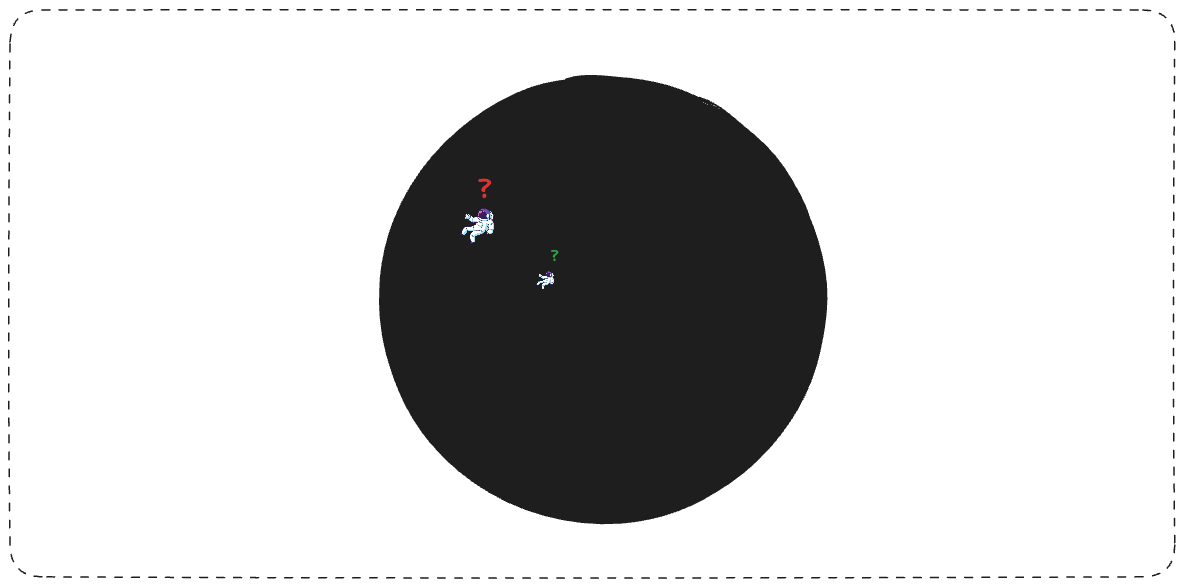
The theoretical answer is that the interior of the black hole is a hologram created by the quantum state of it’s surface. The astronaut exists in both places - because they correspond to one another. It is only one object that manifests in 2 different dimensions.
The 3D interior is a projection from the 2D surface.
In order for this to happen - the astronaut has had to swap time and space.
To see how this is possible you must learn the true nature of a black hole Singularity in Time.
Black Hole Information Paradox
The Black Hole Information Paradox comes about by the idea that information can not be created or destroyed. This is the principle of Unitarity in quantum mechanics. Basically, it means that information about a physical system is never lost.
The evolution of a quantum system is described by a unitary operator which is reversible. If you know any state of a system, you can theoretically determine the initial state.
Hawking Radiation introduced an issue - if objects fall into black holes, and then black holes are evaporated by random quantum fluctuations, then you have lost the information of the objects that fell in. This should be impossible, as information is always conserved.
The Holographic Principle offers a solution to the paradox - all information of objects inside the black hole are contained on it’s surface. When Hawking radiation evaporates the black hole, instead of it being completely random - it is influenced by this surface information, thus conserving it in the radiation itself.