The following explains a phenomenon that shows the simplest form of math will always concentrate wealth to a single holder.
The Con
We often hear that in our world, the unfathomably rich have earned their wealth. They came up with good ideas, worked harder than the rest of us, whatever..
I’d like to show how exactly the same outcome happens when no input whatsoever is applied. You get billionaires through flipping a coin.
A Coin Flip
Through the most basic math, we can become rich! (or 99% of the time, lose all our money) Let’s see how it works.
Let’s imagine that you are in a room with 99 other people, each with $1,000 dollars to start. You are paired with someone, completely randomly, and asked to bet over a coin flip. Let’s say the max people are naturally comfortable wagering is 20% of what they have, so you put down a $200 bet.
You call heads! it’s tails. and you lose.
You are now down to $800. But it’s a game of flipping coins. The odds are 50/50. If we play enough games, your money will obviously even out back to $1000, right?
Here it is, the trick. Let’s play again.
Now you have $800, and you find a new opponent. He happened to win his first game, not that unlikely, 50/50, so he has $1200. You both are only willing to risk 20% of your money again. He bets $240 and you bet $160.
You call tails. The coin is… tails! You win!
Great! You have now won just as many games as you lost! But wait… You have less than the $1,000 you started with. You now only have $960. You only got your bet back.
This was only 2 rounds. What happens when we do, say.. 10,000?
If we simulate 1,000 people flipping a coin 10,000, paired randomly, starting with $1,000 and betting 20% the results are:
Results
Rank Wealth 1 117147 2 39016 3 35976 4 27396 5 19586 6 19252 7 16784 8 15044 9 14610 10 14466 11 13388 12 10544 13 9478 14 9340 15 8942 16 7772 17 7668 18 7637 19 6859 20 6740
This is just one simulation, but it shows that total of $407,645 belongs to just the top 20 players.
This is 40.7% of the wealth belonging to 2% of players through nothing but sheer chance. The top player, representing 0.1% owns 11.7% of the wealth after only 10,000 rounds and 1,000 players.
Extrapolate this to billions of people, and trillions of rounds, and you may see where this is going.
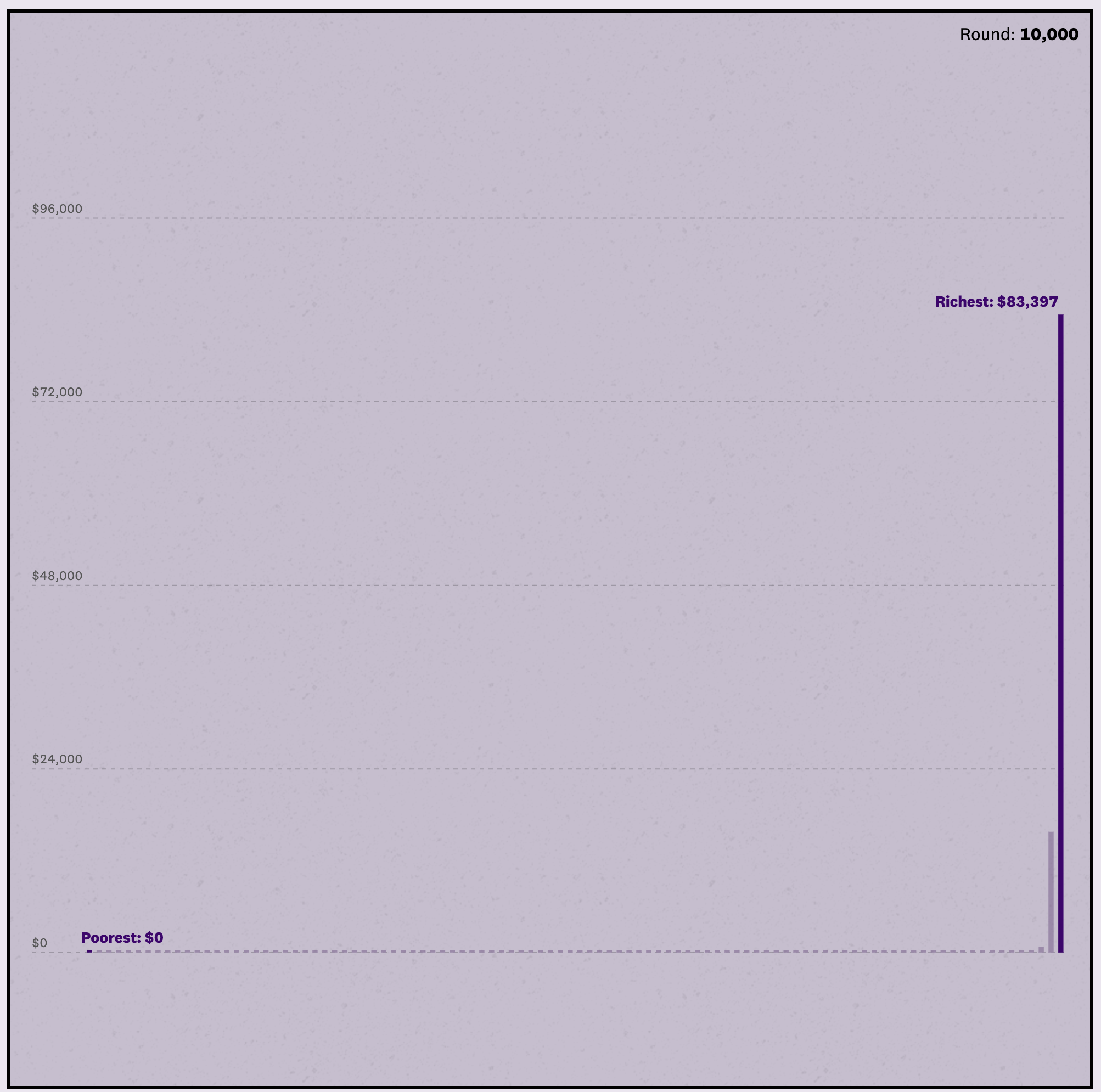
You, by the way, have lost all of your money. You really should have worked harder to be in the 1%.
The Wager
This is counterintuitive. You won half the games. shouldn’t everyone be at $1,000?
This is the simple result of compounding gains.
Compounding means that each round relies on the value of the previous round. This is the driving force behind our capitalist system.
It is not about real value. It is about increasing value relative to the previous rate. Everything must compound.
You’re lead to believe that what you have doesn’t influence what you can do. However, it is everything.
Explainer
- When you lose, the maximum amount you can wager goes down. So you can’t win back what you lost in one coin flip.
- When you win, the maximum amount you can wager goes up. So you could potentially lose more than what you won in the first game.
If you play enough rounds, both players will win about half the games. But the poorer player will lose all of their money.
Let’s start a player with $100 and one with $1,000 and have them coin flip and see what happens.
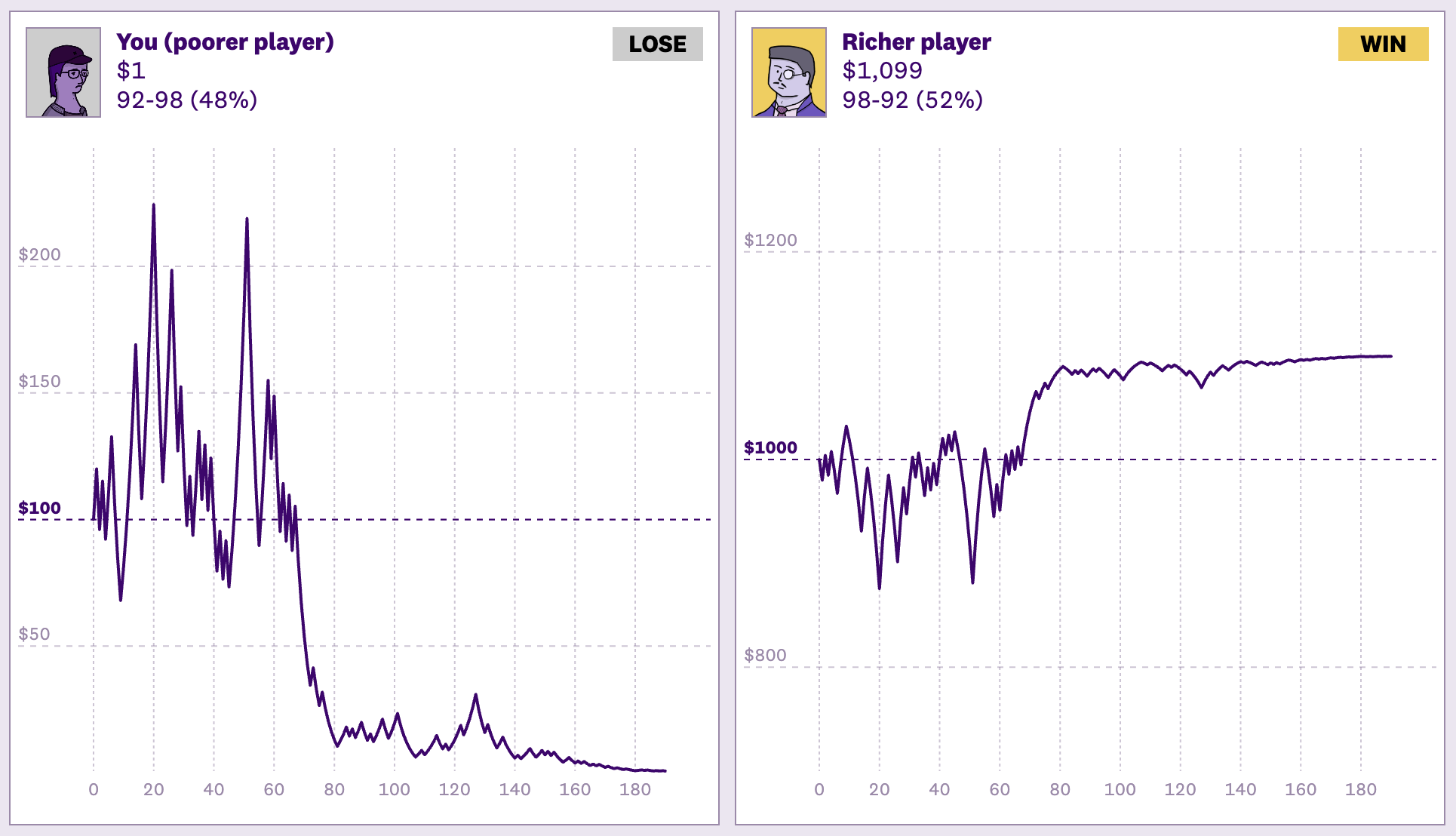
The richer player will get all of the money. That’s because, from their perspective, every game they lose means they have an opportunity to win it back – and then some – in the next coin flip. Every game they win means, no matter what happens in the next coin flip, they’ll still be at a net-plus.
Repeat this process millions of times with millions of people, and you’re left with one insanely rich person.
Redistribution
So is there a way to prevent this pattern? Surely it it doesn’t make any sense to allow a single person to end up with everything through complete luck.
You can apply a redistribution percentage to every round, and it prevents wealth concentration.
If every round we take a small percentage, say 0.5% from everybody and redistribute it equally, the game continues for much longer.
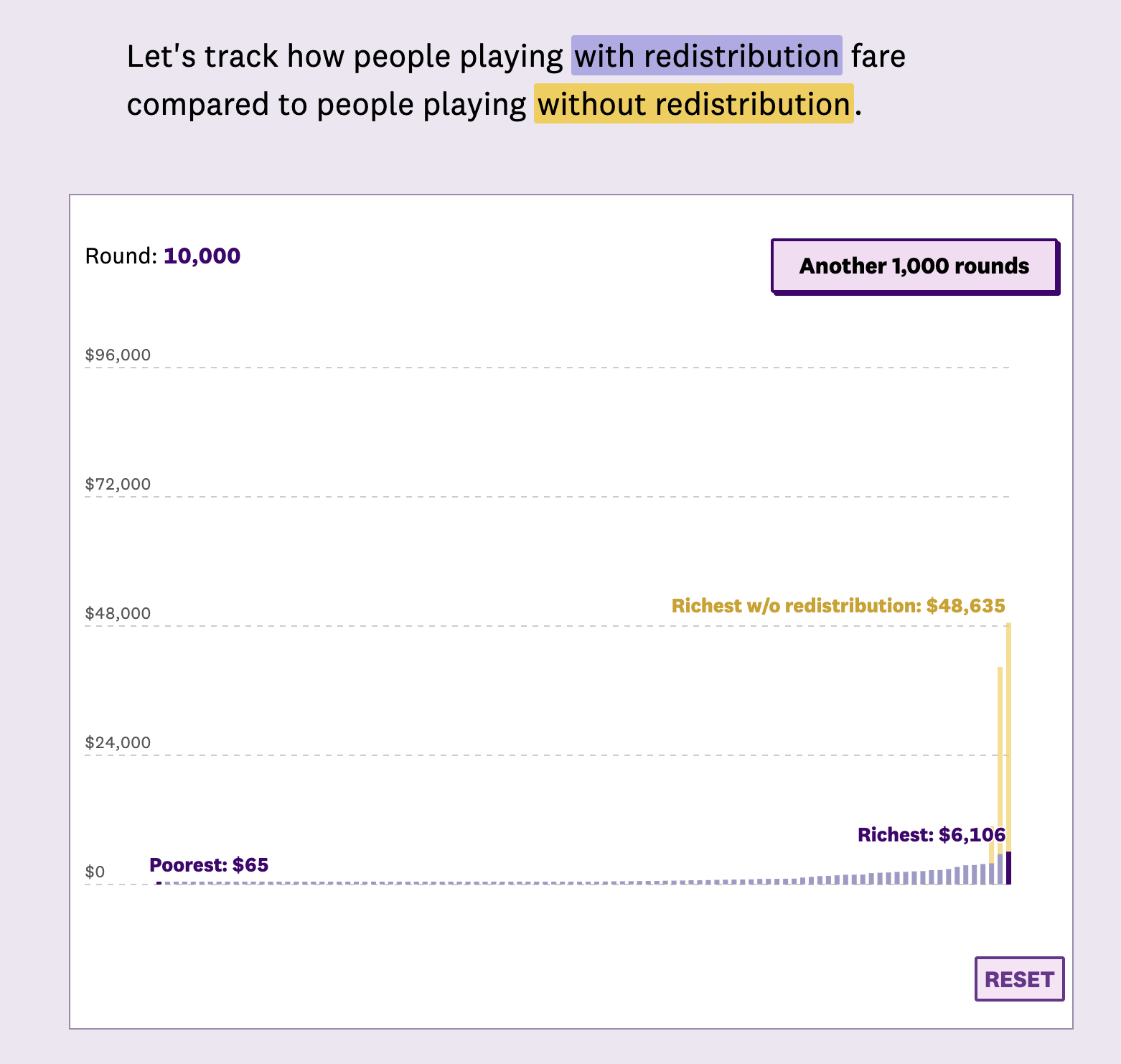
We can see that even a very small amount of redistribution can prevent an uber-wealthy person from emerging.
But even in this model, the richest person is still exponentially more wealthy than the poorest through nothing but pure chance.
Imagine if being rich enabled you to control the outcome of coin flips…
The Real World
In America right now, if the population was represent by 1,000 people, the richest person in the room would have over 4 times the amount of wealth of the poorest 500 people.
But its much worse than that, because america has much more than 1,000 people. Grouping the ultra-wealthy into 0.1% of the population makes their wealth seem tame.
The top 0.1% of the population holds more wealth than the bottom 90% do. This is the exact distribution we’d expect from our coin flip experiment.
These people did not earn this wealth. They have it through sheer chance. And redistribution is the only answer to solve the simple coin flip trick of capitalism.
Citation
This post is directly inspired by pudding.cool, who does a better, more interactive job of telling this story. I just thought it was nice.
If you found this post interesting, read the original there.